One, two, three and away you go. Or should I say one, three, five. If you want to understand how chords derive their notes and names all you need is some systematic thinking and a scale. The numbers game is an essential part of this process and when you finally grasp the idea, it's very enlightening and surprisingly simple.

Our template for this concept is C Major, the mother of all scales;

If you look carefully you'l notice there's only seven different letters. And to see it in text doesn't give you much to go on because it's just an alphabetical series of notes but there's way more there than meets the eye. In fact it's loaded with hidden goodies that affect tons of other musical theories. For this page we'll have a look at a couple of the more pertinant elements.

First we'll get back to the numbers thing. Simply put, if we call 'C' number 1 then 'D' is number 2, 'E' number 3 and likewise on through the rest.

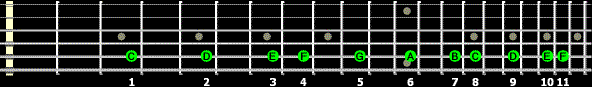
As you can see, if you continue the sequence past 'C' you wind up with higher numbers such as 9, 10, 11 etc. (Ever wonder where those numbers in chords come from?) Nonetheless, you can always consider your first note, in this case 'C', number 1 even if you repeat past it.

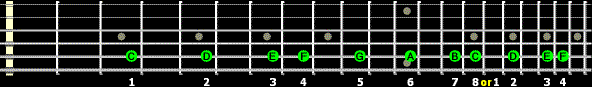
Secondly we have a limited number of notes to deal with. Consider them a family. No matter how you look at it, it'll always be just those notes. And the head of the family, or most important note of all, is 'C'.

Keeping those two things in mind, this leads us to the critical element that drives the whole concept; to manufacture basic chords or triads as they're called, you can count any note in that family as number 1!

Confused? Don't worry, I'll try to clarify.

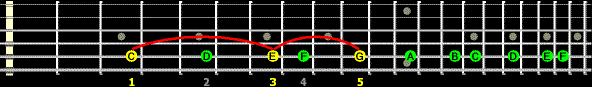
We'll take advantage of a nifty little system involving ones, threes and fives. Once again start on 'C' and call it number 1. From there count up to the third note and that would be ..... 'E'. Continue on and count up to the fifth note, use your fingers if you must, and that's (drum roll please) ..... 'G'! For a grand total of C E G.

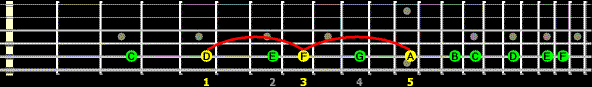
Next, start on 'D' and call that number 1. From 'D' count up to find the third note, 'F', and the fifth, 'A', for a total of D F A.

Now start on 'E' and call that number 1. See a pattern developing here? Count up "a third" to find 'G' and up "a fifth" to find 'B' for E G B.

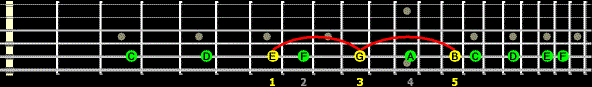
As you go through each note in turn you'll wind up with seven groups of three notes, one for each different letter, and then the process repeats itself. These are the triads which constitute the most basic chords in the key of C. In traditional notation you'd see them arranged like this:

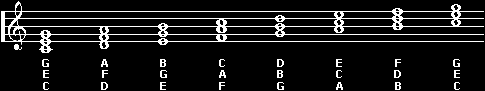
Another cool thing about this system is the chordal value of each group.

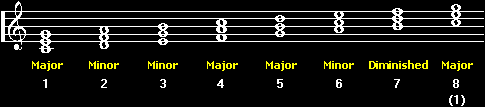
This is a predictable series of chords no matter which major key you work with. In other words, the first or key chord is always major, the second is always minor, the third always minor, the fourth major, fifth major, sixth minor and the seventh chord always diminished. In short: major, minor, minor, major, major, minor, diinished. That's a wonderful thing to be able to take advantage of because it means you don't have to learn an entirely new set of chords for a different key! Just apply the appropriate sharps or flats relevant to the individual notes in that key.

Let's have a look at the key of 'A' Major which goes A B C# D E F# G# A;

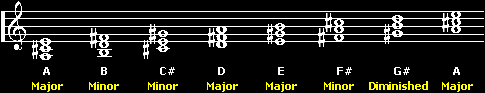
Simply combine the the note name with the chord type and there you have it; A major, B minor, C# minor and so on. It's a beautiful thing to be able to take a simple tool and get a lot of mileage out of it. And that's just one of many!

The question now is 'why are some triads major others minor and what's up with that diminshed thing? And why are they in that particular order?'.

Sorry but the answer to that's a subject for another article on intervals.

It's important to note that this entire process hinges on some very logical, well-thought-out mechanisms. Having a firm grasp of the systems at work and the right tools will eventually let you build or understand anything musical regardless of your instrument.